Previously we’ve discussed the Saaty scale, lets’s see one of its applications using Pairwise Matrix.
In case you missed out on reading about Saaty scale, please refer here. Now, what is a Pairwise matrix? There are two key terms in this word – 1. Pair 2. Matrix
Pair suggests it’s a comparison between two items or two quantities. Matrix is used to represent that comparison. Let’s see how it’s done using an example:
Example:
Let’s say you’re applying for a new job.
And you have chosen 3 parameters – Salary, Domain, Location
- Salary – Just like everyone, Salary is Extremely important to you as well.
- Domain – At this point, you’re just looking for a job, and you’re okay with any domain.
- Location – Bangalore is your favorite location and you’re strongly comfortable with it.
We’re using the same example which was used in the Saaty scale article. Now let’s say you’ve ended up with two job offers.
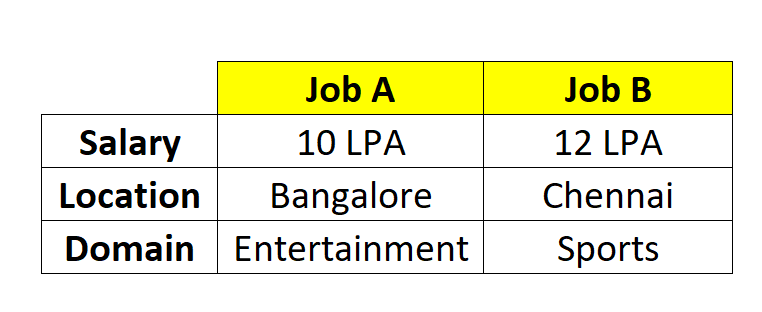
which offer to choose? Let’s solve it using a Pairwise matrix
Step 1: Draw an empty Pairwise matrix
We have to make pairwise comparisons, Salary vs Location, Salary vs Domain, …. and so on
Matrix is the easiest to make such mapping, this is how it looks:
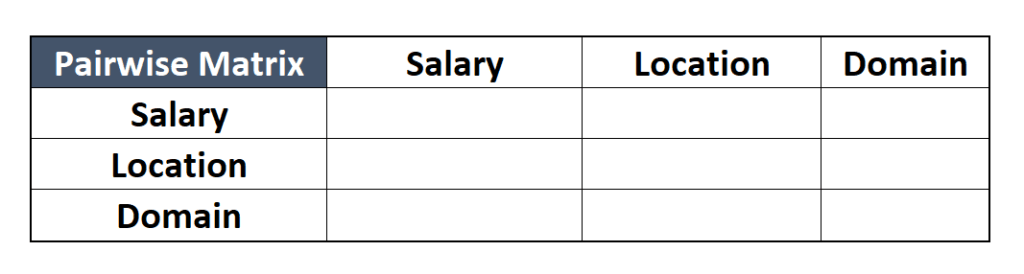
Step 2: Fill up the matrix cells
Now that we have a matrix, we’ll have to fill this up.
Here are the questions you need to ask while filling up the matrix:
Is Salary more important than Location? and vice-versa (Is Location more important than Salary?)
Is Salary more important than Domain? and vice-versa
Is Domain more important than Location? and vice-versa
Because we have 3 variables, we have a total of 6 comparisons (3C2). If we have “n” variables we’ll have nC2 comparisons.
We’ve already discussed that Salary is far more important than Location and Domain, so it’s importance level is 9 (according to Saaty Scale). Let’s fill up these values in the matrix:
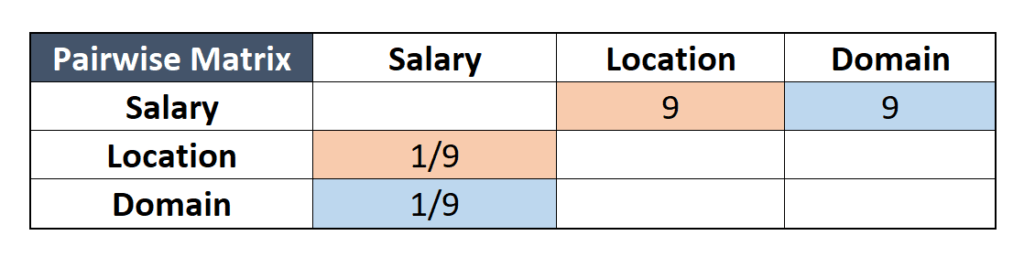
How did this 1/9 come? It’s simple if Salary is 9 times more important than Location (Salary = 9*Location), then Location = (1/9)*Salary.
Next, Location and Domain, we’ve discussed that Location is moderately important compared to Domain. So, the value is 5.
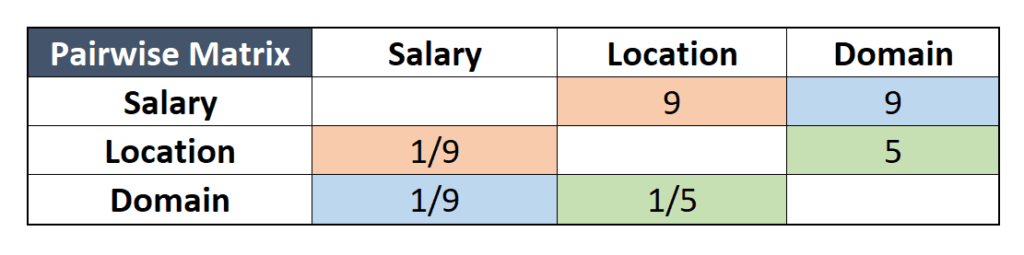
Fill up the diagonal elements with 1 because Salary – Salary, equal importance
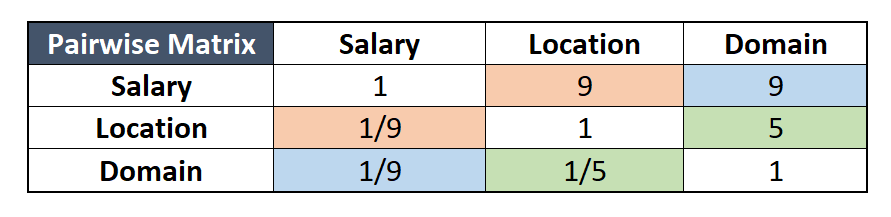
Step 3: Calculate weights in pairwise matrix
Convert fractions into decimals, and then compute the column-wise sum
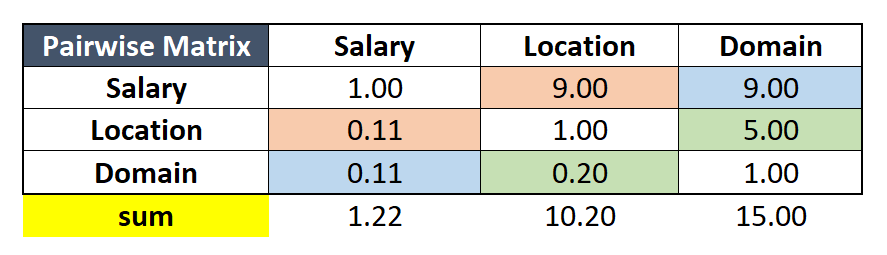
Normalize the matrix by dividing all its col elements by col sum:
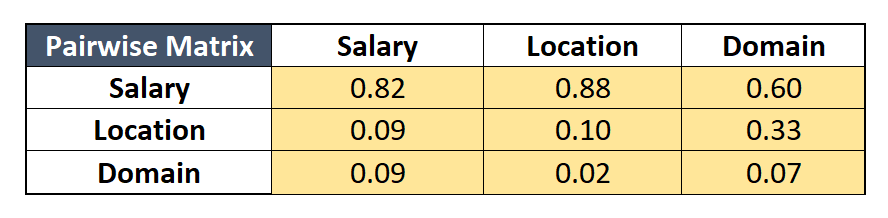
Finally, take the average of each row to get its final weightage
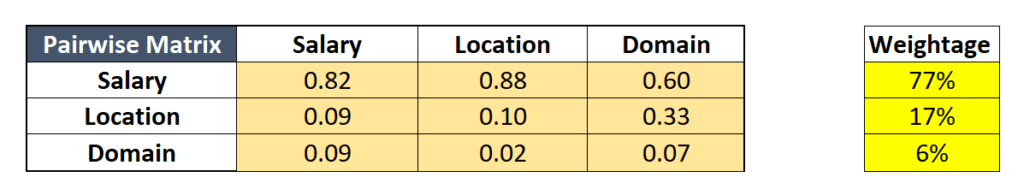
So Salary has 77% weightage, Location – 17% & Domain only 6%.
Clearly, Salary has far more weightage, so you’ll end up choosing Job B.
If you want to get the latest updates on my website about new posts, join the Telegram channel here
